The concept of the time value of money is an essential one for anybody who is involved in the decision-making processes for some critical aspects of finance like capital budgeting and valuation.
On the other hand, it is important for a loan-taker to understand the implications of the time value of money as well.
Simply put, the concept of the time value of money states that a sum of money that is received at this current time, has a higher value than that exact sum of money received down the track in the future.
| Table of Contents |
|---|
| What is Time Value of Money? |
| Components of TVM |
| Time Value of Money Formula |
| Applications of Time Value of Money |
| Key Takeaways |
What is Time Value of Money?
Suppose I offer you the choice of taking Rs. 1,00,000 from me today, or taking this same sum from me after a year.
What decision will you make?
If you chose to take the sum today, you’ve made the right choice.
There are 2 reasons why taking up the first option is better –
High purchasing power – Because of inflation, it’s safe to consider that an amount of money can get us more services and goods than it can in the future.
You have chosen the first option in the previous example because you understand that Rs. 1,00,000 can get you more things today than it will get you a year later.
Risks involved– What if, in the previous example, you chose to receive the money a year later, but when you approach me then, I don’t have any money to give to you?
This could also happen to you if you lend money to someone, but they go bankrupt before they can repay you. This shows that there is a certain level of risk involved if you choose to get your money at a later date.
With these two reasons, we can justify the existence of the concept of the time value of money. Now, let us discuss some components that we’ll need to be able to calculate the time value of money.
Components of TVM
The key components are as mentioned below –
1. Interest/Discount Rate (i)– It’s the rate of discounting or compounding that we apply to an amount of money to calculate its present or future value.
2. Time Periods (n) – It refers to the whole number of time periods for which we want to calculate the present or future value of a sum. These time periods can be annually, semi-annually, quarterly, monthly, weekly, etc.
3. Present value (PV)– The amount of money that we obtain by applying a discounting rate on the future value of any cash flow.
4. Future value (FV)– The amount of money that we obtain by applying a compounding rate on the present value of any cash flow.
5. Installments (PMT)– Installments represent payments to be paid periodically or received during each period. The value is positive when payments have been received and become negative when payments are made.
Time Value of Money Formula
What if you bought a bike and the dealer gives an option to pay Rs 3,00,000, its total cost, now, or 3 installments of Rs 1,00,000 for the next three years at the end of each year.
It’d be wrong if you add the installments and provide a comparison with the current amount that you’ll have to pay.
Why? Let’s find out by learning about the two main calculations that we encounter in situations of time value.
1. Present Value (PV)
The present value is known as the current value of a sum of money that we will receive in the future.
We have mentioned that the purchasing power of money reduces over time. The formula of PV accounts for this reduction by applying a discounting rate to the sum that we will receive in the future.
Learn to Earn Time Value Safely in just 2 hours by Market Experts
Due to the use of the discounting rate, the process of calculating the present value of a sum of money is also known as discounting a sum of money.
The PV of a sum of money can be used to determine the current value of projected cash flow from a bond, an annuity, a loan, or any such instance where you are supposed to receive money from a third party in the future and you want to know exactly how much that money will be worth today.
It is given by the following formula –
PV = FV / (1 + i)^n
Here, we require three things to calculate the present value –
- What is the value of the sum we will receive in the future? (FV);
- What is the rate of discounting at which the purchasing power of the money will fall? (i); and
- After how many years will we receive the concerned sum of money? (n).
2. Future Value (FV)
As the name goes, the FV denotes the value of a sum of money at some date in the future.
This calculation is useful for investors and businesses who want to know the future value of their potential investments to make a good investment decision.
The formula for FV is given by –
FV = PV (1+i)n
This formula requires only three things to give us a future value –
- What amount of money do we have right now? (PV);
- What is the assumed interest rate at which it will grow? (i); and
- After how many years will we need the money? (n)
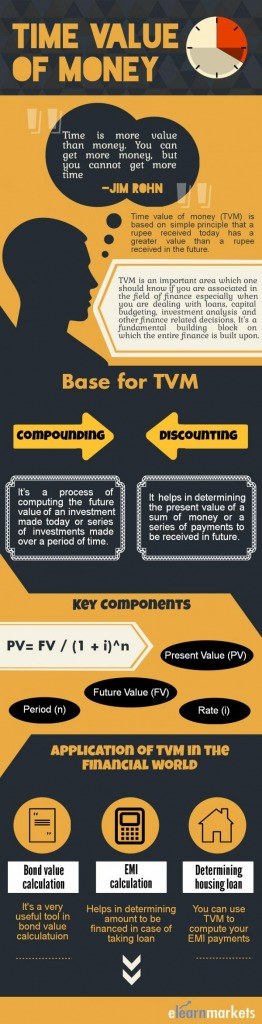
Applications of the time value of money
The concept of the time value of money will be relevant in any situation where a party will be receiving or paying a sum of money to a third party.
That being said, let us discuss two everyday situations where we might have to apply the concepts of the time value of money.
Loan EMIs
EMIs, short for Equated Monthly Installments, constitute the prime method of repaying back a loan.
The need for the concept of time value arises when it comes to calculating the value of the EMIs. Let us return to the example we had talked about before.
As per the example, you went to purchase a bike worth Rs. 3,00,000 and the dealer gives you a choice.
- You either pay Rs 3,00,000 now, or
- Opt for installments of Rs 1,00,000 at the end of each year for the next three years.
Let’s evaluate each choice one by one.
The first one is pretty simple, right? You can just pay off Rs. 3,00,000 this instant, but this amount can cause a huge dent in your savings, depending on your income and financial status.
Now, for the second one, if you pay Rs. 1,00,000 at the end of each year for three years, it might cause you a bigger dent in your savings because as we’ve learned, the value of money keeps increasing over time.
Therefore, it is clear that even if we are paying three installments of Rs. 1,00,000 each, the effective value of these installments can be much higher in the future.
It’s because the installments are not the present values, whereas the Rs. 3,00,000 is the present value of your purchase.
One should always make sure that they are either comparing two future values or two current values to come to a correct investment decision.
Therefore, to make the correct decision, you should evaluate the present value of all the installments, add up their value, and then compare that value to the current price of the product to see how much interest you’re paying on the product.
This technique is relevant for any loan – from a home loan to a simple EMI-system purchase of home appliances. You can read about calculating EMIs in an easy way.
Value of Investments in Bonds
The PV formula plays a pivotal role when it comes to calculating the yield to maturity (YTM) of a bond.
What is the Yield to Maturity?
In simple terms, the YTM is the rate of return that shows the total anticipated return to an investor if they hold the bond till maturity.
Consider this – suppose an investor wants to invest in a security that will yield them an interest rate of at least 10%.
How will the investor be able to decide if the bonds they want to purchase will give them as many returns?
Simple! Calculating the YTM requires the calculation of the PV of every cash flow from the bond to the investor.
Therefore, the investor can compare the YTM rate with their desired rate of interest to decide if the bonds are a suitable purchase for them.
In this way, the concept of time value is essential for an investor looking to buy bonds in the future.
You can also read more about YTM and bond yields.
Key Takeaways –
- Time value of money is an important concept which one should understand if they are associated with the fields of finance.
- Time value of money works on the principle that money today is worth more than the same amount of money received in the future.
- There are 5 major components of time value – rates, time periods, present value, future value, and payments.
- The Present Value (PV) is known as the current value of a sum of money that we will receive in the future.
- The Future Value (FV) denotes the value of a sum of money at some date in the future.
- The concepts of present and future value are essential for many day-to-day situations involving the exchange of money.






I found your article very informative and you had great use of facts throughout the article.
Thanks for sharing this valuable information with us, it is a really helpful article!
Hi Jessie,
Thank you for Reading!
Keep Reading!
Your article is very informative and very helpful about this topic thanks for share this informative article.
Hi,
We really appreciated that you liked our blog! However, there is no option to download the same.
Thank you for Reading!
Keep Reading!